Master's thesis defense meeting (Mr. Abbas Haji-Heidari)
Title: Adjoin-based optimization of three-dimensional wavy wings at design and off-design conditions
Presenter: Abbas Haji-Heidari Vernosfaderani
Supervisors: Dr.Mahdi Nili-Ahmadabadi
Reviewers: Dr. Ahmad Reza Pishevar – Dr. Mohammad Reza Tavakoli
Time and Place: November, 19, 2024, Time: 8:00 AM, Location: Council Room, Faculty of Mechanical Engineering
Abstract
Geometric optimization in aerodynamics aims to improve the performance and efficiency of fluid systems. Selecting an appropriate optimization method is crucial, as it directly impacts computational cost and the accuracy of results. This study employs the adjoint method, a gradient-based approach, for optimization. Gradient-based methods use the derivative of the objective function concerning design variables to find local optima with high accuracy. The advantage of the adjoint method lies in its computational cost being independent of the number of design variables, making it particularly suitable for geometric optimization problems with many variables.
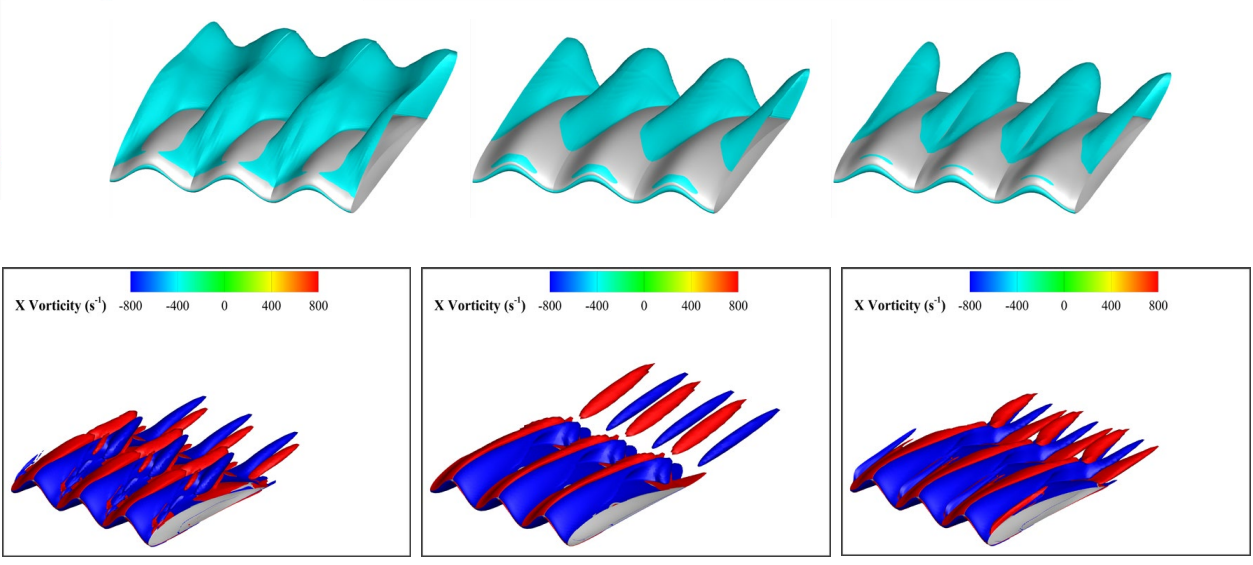
In this research, the adjoint module in ANSYS Fluent was used to optimize the aerodynamics of a three-dimensional wavy wing, with the Bézier curve applied for geometry parameterization. Numerical solutions for the governing flow equations were computed using ANSYS Fluent. The tubercles on humpback whale flippers inspired the concept of introducing wavy protuberances along the wing’s leading edge. In this research, the wavy contour extends from the leading edge to the trailing edge along the chord.
Three wavy wings with symmetric and asymmetric airfoil cross-sections were created, and the wavy profile along the chord was optimized to enhance the three-dimensional flow field around the wing. Two objective functions were considered: increasing the lift-to-drag ratio and reducing drag while maintaining a specific lift coefficient. All surface nodes of the wing were treated as design variables. To reduce computational costs, only a single wavelength of the three-dimensional wavy wing was simulated using periodic boundary conditions.
In the first part, the optimization was applied to two wavy wings with a 2° angular amplitude, featuring symmetric and asymmetric airfoil sections. The optimization process increased the lift-to-drag ratio by 66% and 11%, respectively, leading to significant performance improvements, both pre-stall and post-stall. Additionally, the stall angle for the symmetric airfoil wing was delayed by 1 degree.
In the second part, optimization was performed on a wavy wing with a 6.5° angular amplitude and an asymmetric airfoil section. Considering both design and off-design conditions (stall conditions), the optimization process used the two objective functions. The design-point results showed that the first and second objective functions improved by 21.1% and decreased by 12%, respectively. In off-design conditions, the objectives improved by 15.38% and 26.5%, respectively. The comparison of performance curves between optimized geometries indicated that the first objective function is more suitable for design-point optimization, while the second is preferable for off-design conditions.
The optimization results for all three wings revealed localized protrusions on the suction surface, controlling pressure gradients and flow separation. Furthermore, localized depressions near the trailing edge on the pressure surface increased wing loading and lift coefficient. A comparison of flow fields behind the baseline and optimized wings indicated a reduction in the size and spacing of the clockwise and counterclockwise vortices in the wing’s wake, with an increase in vorticity. Finally, comparing the optimized wings showed that the final wing with a 2-degree wave amplitude and an asymmetric airfoil cross-section achieved the highest aerodynamic efficiency.
Keywords:
Optimization, Adjoint method, Aerodynamics, Wavy wing, Computational fluid dynamics, Lift-to-drag ratio, Drag reduction with fixed lift